
The risk of transmission will be affected by the ventilation rate and the type of respiratory activity, as well as exposure time
Although the near field (<2m) transmission risk is well established via droplet transmission, many scientists have warned about the possibility of indoor far field (>2m) transmission of the SARS-CoV-2 virus encapsulated in small respiratory aerosols that can remain airborne for several hours.1 Building services engineering bodies, such as CIBSE, have responded by encouraging improved ventilation strategies to reduce transmission risk.
Poorly ventilated indoor spaces are at an increased risk of SARS-CoV-2 transmission, and several methods have been proposed to assess the risk indoors. One challenge in developing a transmission-risk methodology is that the required dose of SARS-CoV-2 to produce an infection is unknown, and the amount of virus per millilitre of respiratory fluid that an infected individual may shed ranges over several orders of magnitude.2,3
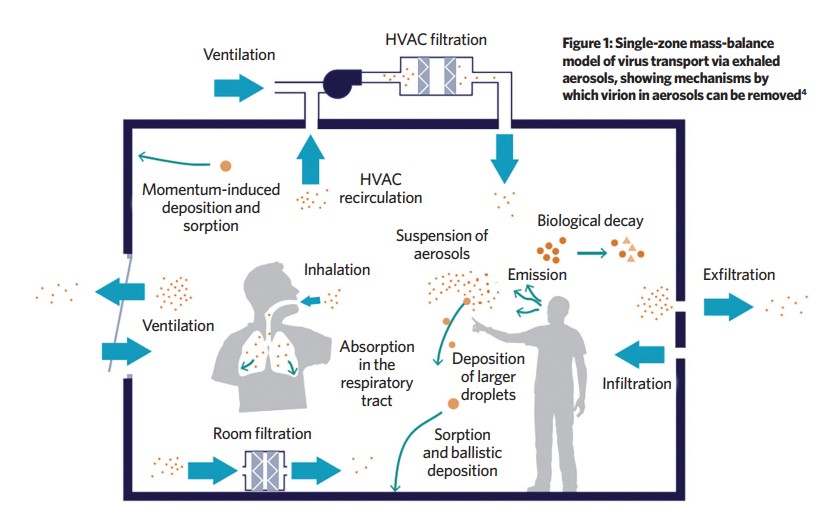
The methodology is described in the pre-print paper Modelling uncertainty in the relative risk of exposure to the SARS-CoV-2 virus by airborne aerosol transmission in buildings by Benjamin Jones, Patrick Sharpe, Chris Iddon, Abigail Hathway and Shaun Fitzgerald. In the paper, a dose risk for a reference space is established and the dose risk of another space can then be compared to generate a relative risk index (RRI) for aerosol transmission, such that any uncertainty in the viral load of infector – or on the viral load required for infection – cancel out.4 The method establishes how risky a space/activity is compared with the reference case. It can be used to establish the effectiveness of mitigation measures in reducing RRI.
It is important to appreciate that this model only considers the far-field transmission of SARS-CoV-2 by aerosol in a well-mixed space, and does not consider the near field (<2m) or fomite transmission. The concentration of SARS-CoV-2-laden aerosols from an infected individual will be greater within the exhaled puff than in the well-mixed room.5 Social distancing is, therefore, still required and, if you are close to an individual and the infectious material has not become well mixed, transmission risk will be higher. In spaces where social distancing is not possible, consideration of droplet spread and appropriate PPE is required.
The model imagines an infected individual emitting viral-laden aerosols (VLA) as a pollution source and assumes a well-mixed space. With no dilution mechanisms, the number of VLA in the space would increase linearly over time. However, there are several ways in which the VLA are removed from a space – or rendered inactive (see Figure 1):
- Ventilation
- Ballistic and momentum-induced deposition removes VLA as they become adsorbed onto surfacesBiological decay of the entrained virus, becoming inactive over time
- Removal by filtration
- Inhalation by other occupants.
It is challenging to establish how many viral particles (virions) may be emitted by infected individuals. However, it is possible to measure the genetic material of the virus using the laboratory method quantitative reverse transcriptase polymerase chain reaction (RT-PCR), where fragments of the viral genome in a sample are amplified via a biological replication method and then quantified.
These genomic fragments of RNA do not give a value for the number of infective virion present – merely a measure of genomic material, much of which may be fragments of decayed virion. Other respiratory viruses studies suggest the ratio of infective virion to RNA copies is in the order of 1:100 and 1:1000.6
There are published measures of the SARS-CoV-2 genomic material in the respiratory fluid of infected individuals and the air of rooms housing infected patients. Therefore, this method has used ‘copies of RNA’ emitted by an infector, on the basis that an unknown portion of this genomic material is likely to be infective virion.
The number of RNA copies emitted by an infected individual into the space – G – is diluted by a number of mechanisms, described above, that can be normalised by the volume of the space – V (m3) – and combined into a single dilution rate – φ (s-1) – by addition.
The equations for the number of RNA copies inhaled by a susceptible individual in a scenario where an infector enters the room at time = 0 (novel), or if a susceptible person enters a room where an infector has been present for sufficient time that the number of RNA copies within the space has reached steady state, are then derived (see ‘Equations for number of copies of RNA inhaled’ panel).
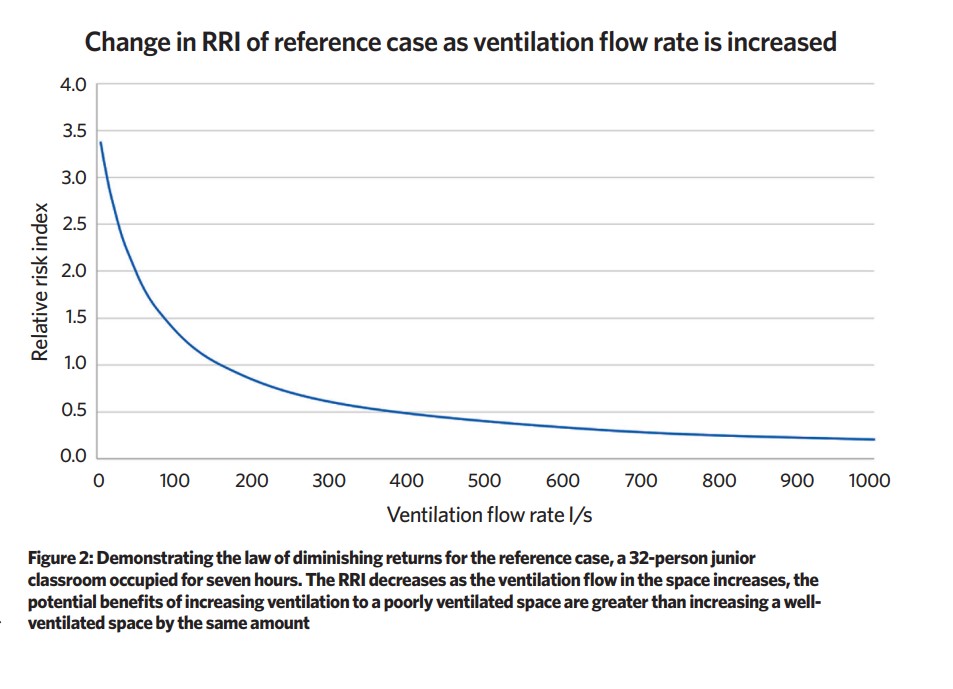
Ventilation plays a key role in dilution – poorly ventilated spaces increase RRI, although there is a diminishing law of returns in increasing the ventilation rate; the potential benefits of increasing ventilation to a poorly ventilated space are greater than increasing a well-ventilated space by the same amount
There are ranges of uncertainty for many of the inputs and, in the paper, a statistical framework to estimate uncertainty of the number of SARS-CoV-2 RNA copies inhaled is employed. The reference space is a junior classroom occupied for seven hours with a ventilation rate of 5 L·s-1 per person; the form and ventilation rates for these spaces are well defined in current Department for Education (DfE) guidelines and the paper describes the reference case and assumptions fully.7,8
In 2009, Morawska et al undertook several experiments to establish the amount of aerosols produced by an individual under various respiratory activities (for example, breathing, talking and vocalisation), so it is possible to derive a volume of respiratory fluid released per m3 of exhaled breath for various respiratory activities.9
The total number of RNA copies released into the air by an infected individual is a function of the breathing rate, respiratory activity and the concentration of RNA copies per ml of respiratory fluid of the infected individual, providing the emission rate kqsus G. The analysis suggests that the volume of respiratory fluid released as aerosols is roughly 1:5:30 for breathing, speaking and vocalisation respectively.

Vocalisation activities should be minimised unless there are elevated levels of ventilation per person
The mathematics of the model demonstrate that the RRI for aerosol transmission is most sensitive to the emission rate (G), so steps to reduce G should be considered (respiratory activities that produce more aerosols – such as vocalisation – should be minimised unless there are elevated levels of ventilation per person; face coverings to reduce G should be encouraged).
Ventilation plays a key role in dilution – poorly ventilated spaces (for example, infiltration only) increase RRI, although there is a diminishing law of returns in increasing the ventilation rate (see Figure 2); the potential benefits of increasing ventilation to a poorly ventilated space are greater than increasing a well-ventilated space by the same amount.
The model only considers the far-field risk of infection in a well-mixed room, and helps engineers reduce this risk. Social distancing and hand washing are still essential. CJ
For more analysis and a spreadsheet tool see the preprint at ResearchGate via or email naturalventilation@cibse.org
About the author
Chris Iddon is chair of the CIBSE Natural Ventilation Group
References:
1 Fennelly, K P, Viewpoint: Particle sizes of infectious aerosols: implications for infection control. Lancet Respir. 2600, 1–11 (2020).
2 Wölfel, R et al, Virological assessment of hospitalised patients with Covid-2019. Nature 581, (2020).
3 Miller, S L et al, Transmission of SARS-CoV-2 by inhalation of respiratory aerosol in the Skagit Valley Chorale superspreading event. MedRxiv Prepr. 1–17 (2020)
4 Jones, B, Sharpe, P, Iddon, C, Fitzgerald, S, & Abigail, E, Modelling uncertainty in the relative risk of exposure to the SARS-CoV-2 virus by airborne aerosol transmission in buildings. Prepr. Res. Gate (2020)
5 Bourouiba, L, Turbulent gas clouds and respiratory pathogen emissions: potential implications for reducing transmission of Covid-19. JAMA –
J. Am. Med. Assoc. 323, 1837–1838 (2020).
6 Yan, J et al, Infectious virus in exhaled breath of symptomatic seasonal influenza cases from a college community. Proc. Natl. Acad. Sci. U. S. A. 115, 1081–1086 (2018).
7 DfE. BB103 Area guidelines for mainstream schools. Sch. Build. Des. Maint. 39–40 (2014).
8 Education and Skills Funding Agency. Building Bulletin 101 Guidance on ventilation, thermal comfort and indoor air quality in schools. (2018).
9 Morawska, L et al, Size distribution and sites of origin of droplets expelled from the human respiratory tract during expiratory activities. J. Aerosol Sci. 40, 256–269 (2009).
